“To Count” can mean two things.
The first meaning is to point to a thing and say the number 1, then point to the next thing and say the number 2, then point to the next thing and say the next number, and continue doing this until all the things have been pointed to and labeled with a number.
The second meaning is to be important.
A lot of math can be performed by counting. Now bear with me while I explain how.
To add two numbers you can go out and gather a bunch of things, hopefully easy to pick up, and count out a group with the first number of things, then count out a second group with the second number of things, then combine the two groups and count the combined group’s number of things.
To subtract two numbers you can count out a group with the first number, then, out of that first group, count out a second group with the second number. After taking the second group away, count how many remain in the first group.
To multiply two numbers you can count out a group of the first number, then make more groups of the same number, counting out the second number of groups. Then combine the groups and count the combined group’s number of things.
To divide two numbers you can count out a group of the first number, then start dealing out the things like a deck of cards among the second number of groups. Once you run out of things to deal out, you count how many things are in each group.
The reason I have explained how to use counting to do math is because to me, it was hard-won information. I went to a public school that did a little experiment in my class, called Open Classroom. The principal was very much against this type of classroom, but boy did I love it. To this day, it is my memory of my favorite study environment. Open classroom means you as a student can choose what area of the room you want to be in – whether the reading carpet area surrounded by book cases (oh yeah), the workshop corner (where you could only go to on Thursdays when a part-time teacher would be there ready to help you saw wood), the science area where sometimes a teacher would be available to let you play with the guinea pig or to poke at the monarch caterpillar cocoons if they were in season, the picnic table on the other side of the bookcases used as the writing area, or the other picnic table on the other side of room used as the math area.
Now here is where I will make a little observation of open classroom. If a six-year old in an open classroom doesn’t understand the teaching methods used to teach a subject, that six-year old will avoid those teaching methods in the easiest way made available – by avoiding the subject. So, if you are going to be doing some experimental type of classroom like Open Classroom, you better also allow choices for how to learn a subject, not just choice as to whether or not to learn the subject.
Alas, the math picnic table did not offer me the option to learn how to do math by counting. The math picnic table instead only provided one teaching method for math: Cuisenaire rods. Cuisenaire rods are these awesome rods that are like measuring sticks with no markings. There are ten different sized rods, ranging from 1 centimeter to 10 centimeters long. Each length is a different color (1 centimeter rods are always white, 2 centimeter rod are always red, etc.).

Every kid in existence is able to understand math from using Cuisenaire rods. Except for me.
At the top of this blog is a picture from my notebook from a day when I have wandered over to the math picnic table. The date is September 15. It is still relatively early in the school year. I have spent the first two blissful weeks of school on the carpeted reading area, doing nothing but reading. Today I hear that there is a teacher in the woodworking area, so I decide to explore something new. I do some dangerous stuff in that dark and mysterious woodworking corner, then I try my hand at writing composition, then I check out the Guinea pigs. Next to the Guinea pigs is the math picnic table. For whatever reason, I go sit down there, acutely aware that the students sitting there probably have a two week head-start on whatever it is that is being explained here. I distinctly remember writing down what the teacher was demonstrating, and leaving the picnic table completely baffled. How are two sticks equal to 19? What is 19? Do I have to memorize which color combinations make 19? I decide not to visit the math picnic table anymore. I do not learn how to add. I certainly do not learn how to subtract. And when my parents decide to move to a different state in the spring of that school year, I enter a new public school and am given a subtraction test.
I am staring at the subtraction test and I realize I have never learned subtraction. I hide my hands under the desk and start using my fingers, and I am ashamed. I am trying to figure out how to do subtraction all by myself. When I was very young, before I ever went to school, I remember my older brother bragging that he had figured out how to use his fingers to add – figured it out all on his own – before he ever entered school. I must have been four at the time, and I had never added on my own fingers – I had never seen the need for it – and I figured that I would be taught how to do so when I entered school. Well, by age six sure enough I did see the method here and there from friends, along with the ridicule that it is a baby method. I knew nobody else doing the math test was using their fingers – I had the sense that it was against the rules.
Which brings me to the second definition of “to count.” Importance. Why were some Cuisenaire rods of different lengths and colors more important than others, so that they counted more? Why was using my fingers to subtract so forbidden? Why was math so mean? It made me ashamed. And why did it have to throw so many numbers at me, like weapons or threats?
To illustrate why so many of us have a fear of math, I would like to steal from material from a Ted Talk given by Dan Meyers.
He gives the example of a math problem:
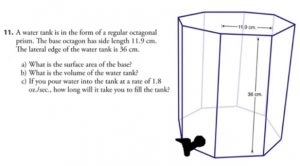
And then he gets rid of the numbers. The only writing that remains is: A water tank… how long will it take you to fill the tank?
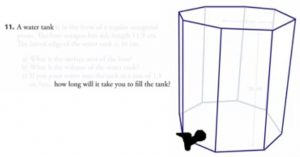
And then he gets ready to count the minutes:

But counting is tedious. So as the students are waiting for the tank to fill up, he asks them for their guesses.
And guesses are something that anyone can engage in. Suddenly, the students who are intimidated by numbers are on the same level playing field as the rest of the class. So for the first time, these students start participating. And as the discussion opens up, suddenly the students start realizing that they could guess a lot better if they had more information: how tall is the tank? How wide around is it? How fast is the water flowing? And only then, when they ask for the numbers, are they given them.
So the second definition of to count is also an impediment. When too many things are made important, stressed, and it is not clear why those things are important, a person feels overwhelmed. You would think, that after all my ranting about counting, that I would say counting is the way to teach math. But really, the irony is that counting isn’t important in teaching math either.
When I was 23 I got a job teaching two year-olds. Part of the curriculum was to teach them to “recognize quantities up to 10.” I started out by teaching them to count, but the headmistress of the school took me to the side and said, “no.” She told me that I was to teach them to recognize quantities BEFORE I taught them how to count. She said that the mainstream way to do is wrong – as in backwards – as in kids are always first taught to count in order to recognize quantities. But kids can recognize that two objects are two objects just by looking at them, she argued – they don’t need to count to do that. Same with three objects, same with four. Get the kids to recognize objects up to 10 BEFORE they learn how to count. Teach them the word for larger and larger amounts of objects, but don’t point out each one, labeling each one with a number to get to the final number. Just tell them: this is 5. This is 6. See if they can tell you how many they see. The kids that she taught to really get a sense of how many or few items were in front of them, she said, never had trouble with math in the future.
I have to agree that what she said makes a lot of sense. If you ever watched a two year-old or even a six year-old count, it is a painful thing. They don’t do it methodically, they point to objects but don’t think of moving or grouping or indicating they have counted the objects, so that they inevitably double count or skip an object. On top of that, they often forget what number they are on or don’t know the next number, or go from seventeen to seventy. If they only had a sense of how large or small a number was, they would know they didn’t have seventy objects when they really had seventeen: it just wouldn’t “look” right. Also, if they knew what five objects looked like, they would know in their bones that there is a group of two plus a group of three that make the “look” of a group of five.
Now I will make a confession – I never did get a two year old to recognize more than four objects by glancing at them, nor did I get a two year old who could maintain an attention span long enough to practice the skill. I invented the “Duplo-castle game” where they had to guess the right number of Duplos placed in front of them in order to be able to take them to make a castle. However, a two-year old will tolerate such a game for only so long before realizing that there are many times and other places to access Duplos and play with them that don’t require jumping through such hoops. Five year-olds do a lot better in guessing quantities solely for the challenge of it, though they usually cheat by counting, since they are so determined to give the right answer, and not be wrong.
It is a hard lesson to teach someone that guessing is a skill, and that performing mindless functions to get the right answer is not a skill. A calculator will allow you to give the right answer. But knowing when the calculator’s answer is ridiculous and to doubt it enough to investigate if there was a user entry error or a program bug – that is a skill.
So many clients are scared to do accounting or fill out government forms. It has to do with the basic fear of counting. Fear of adding wrong, subtracting wrong, multiplying and dividing wrong. (Sorry, there isn’t any higher math in accounting that is needed than these four functions.) Even though calculators can do these four functions, clients are simply scared of the numbers. I know what it feels like to have numbers thrown at me, to have methods thrown at me that made no sense, to have methods forbidden that were my only grasp on sanity.
When looking at a government form, the importance of the form is not given, the rational for each question is not given. It takes years of accounting classes before understanding the government’s problems, their thought-process, their needs, and why they ask what they ask and calculate they way they calculate. Their questions can be ambiguous – and the punishment for misinterpreting their question the wrong way can seem unfair. It certainly creates job security for accountants, who have learned throughout the years how to interpret the question.
